 I am interested in shape, dynamics, and mechanics. Often this leads me to problems involving curved thin structures such as chains, strings, rods, membranes, plates, and shells. My group spends much of its time deriving and solving differential equations and playing in the lab.
Much of our recent, current, and planned activity falls into one of the following overlapping areas:
I am interested in shape, dynamics, and mechanics. Often this leads me to problems involving curved thin structures such as chains, strings, rods, membranes, plates, and shells. My group spends much of its time deriving and solving differential equations and playing in the lab.
Much of our recent, current, and planned activity falls into one of the following overlapping areas:
-
Elasticity , including wrinkle-roller interaction in sheet processing, the behavior of incompatible continua and structures, multi-stability and bifurcation, and the stretching and bending of surfaces. How do elastic "defects" interact, form patterns, and mediate snap-buckling in plates and shells? What are the simplest bending energies for shells? -
Discontinuities, variational principles,
pseudomomentum , and material symmetry, including developing tools based on balance laws and (non)conserved quantities in (in)homogeneous media, and the counterintuitive physics of moving contacts between flexible and rigid bodies. When can we apply action principles to variable-mass problems such as water entry, popping balloons, or the cutting and winding of sheets? -
Dynamics of flexible bodies , including their interaction with fluid, and their relative equilibria subject to inertia, drag, and other forces. What shapes are adopted by a towed cable or by unspooling yarn? How does energy localize when a cable is pulled? -
Selective modification of dynamical systems. Can we use recently developed tools to find structure in complicated flows or create new integrable systems?
Here is an

- Buckling mediated by mobile localized elastic excitations
Hutton, Vitral, Hamm, Hanna
[arXiv:2310.17478] | PNAS Nexus 3 (4) pgae083 (2024) - Crumple dynamics
Hutton, Vitral, Hanna, Hamm
Gallery of Soft Matter submission 2022
Transient and stable patterns and particle-like behavior of localized curvature.
- Momentum and pseudomomentum in a shallow water equation
Hanna
[arXiv:2208.05657] | Physics of Fluids 34 (11) 116603 (2022)
Wading into this topic for the first time. A preliminary exercise, hoping to eventually relate two approaches (see below for long paper on pseudomomentum on which this is based).
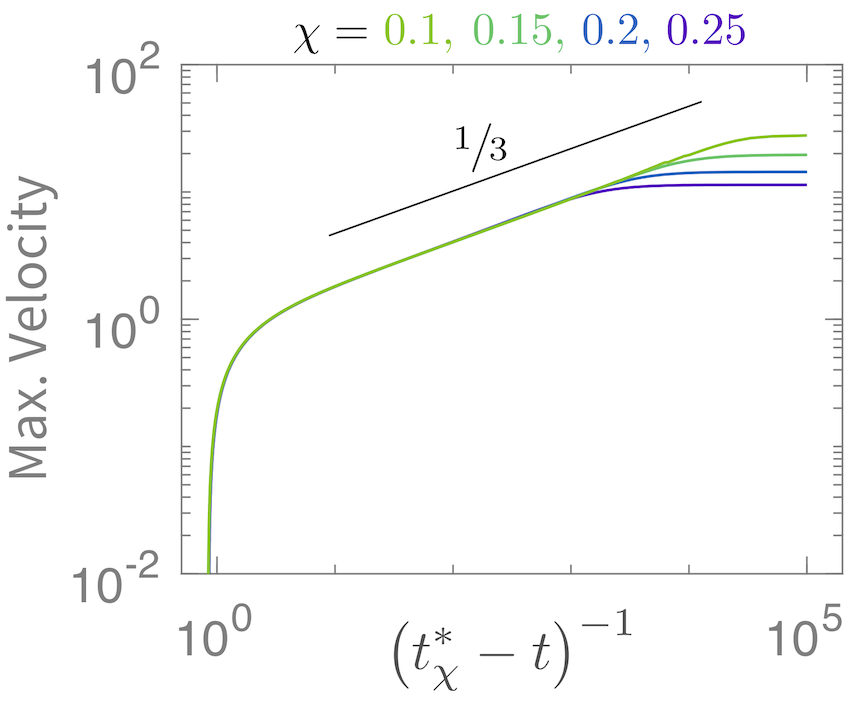
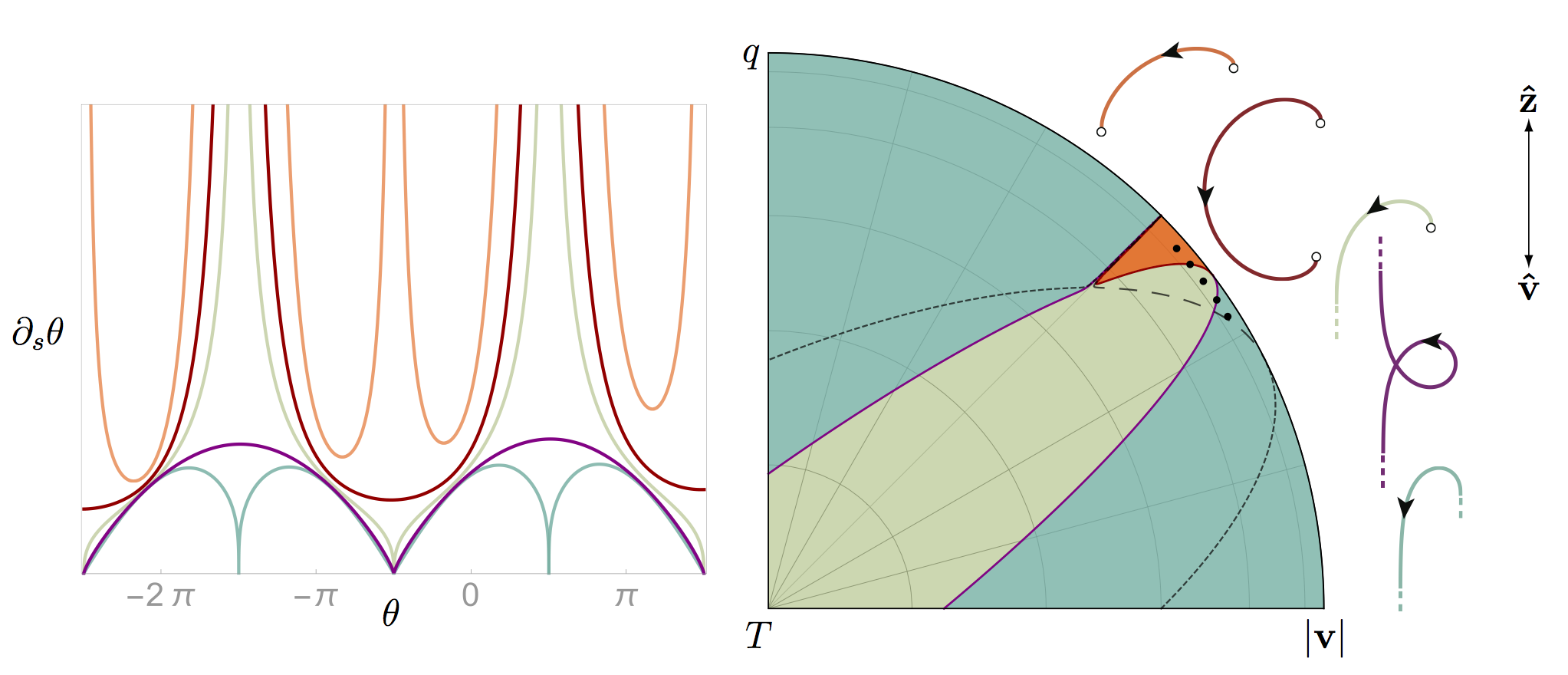
- Anomalous curvature evolution and geometric regularization of energy focusing in the snapping dynamics of a flexible body
Dehadrai, Hanna
[arXiv:2206.07864] | Mathematics and Mechanics of Solids 29 (6) 1125-1135 (2024)
The snapping (whipping, flapping) singularity and its regularization.
- Energies for elastic plates and shells from quadratic-stretch elasticity
Vitral, Hanna
[arXiv:2201.05608] | Journal of Elasticity 153 (4-5) 581-598 (2023) - Dilation-invariant bending of elastic plates, and broken symmetry in shells
Vitral, Hanna
[arXiv:2111.01349] | Journal of Elasticity 153 (4-5) 571-579 (2023)
Two complementary routes to obtain primitive bending measures and energies for plates and shells. The plate measure is a generalization of an old result for straight rods, while the shell measure and its restriction to curved rods is entirely new. We compare with various commonly used models in soft matter, which all behave in qualitatively different ways.
- Quadratic-stretch elasticity
Vitral, Hanna
[arXiv:2104.11714] | Mathematics and Mechanics of Solids 27 (3) 462-473 (2022)
A first stab at developing a systematic small-stretch elasticity theory, with an eye towards plate and shell theories.
- Exterior dissipation, proportional decay, and integrals of motion
Aureli, Hanna
[arXiv:2103.15173] | Physical Review Letters 127 134101 (2021) - An integrable family of torqued, damped, rigid rotors
Hanna
[arXiv:2007.00707] | Mechanics Research Communications 116 103768 (2021)
The first note on rigid body rotation started as a homework problem but got a bit more interesting. The follow up is a significant generalization in which we construct modifications to dynamical systems that selectively change their conserved quantities while retaining as much integrability as possible, driving the system towards nontrivial states.

- Cutting holes in bistable folds
Yu, Andrade-Silva, Dias, Hanna
[arXiv:2008.12382] | Mechanics Research Communications 124 103700 (2022)
Exploring how the excision of singularities, creating virtual singularities, affects stiffness and multistability in sheets. In the case studied here, there is a curious re-entrant dependence on hole shape parameters.

- Anisotropic swelling of anisotropic elastic panels
Wood, Hanna
[arXiv:2008.06166] | Soft Matter 17 3137-3143 (2021)
Plywood meets embeddings. A triumph of nominative determinism (see also bendy straw paper below).
- Pseudomomentum: origins and consequences
Singh, Hanna
[arXiv:2007.06023] | Zeitschrift für angewandte Mathematik und Physik 72 122 (2021) and Correction 73 189 (2022)
Exploration of a balance law connected to material symmetry, with examples from elasticity, fluids, and structures. Corrects informal approaches in my previous papers.
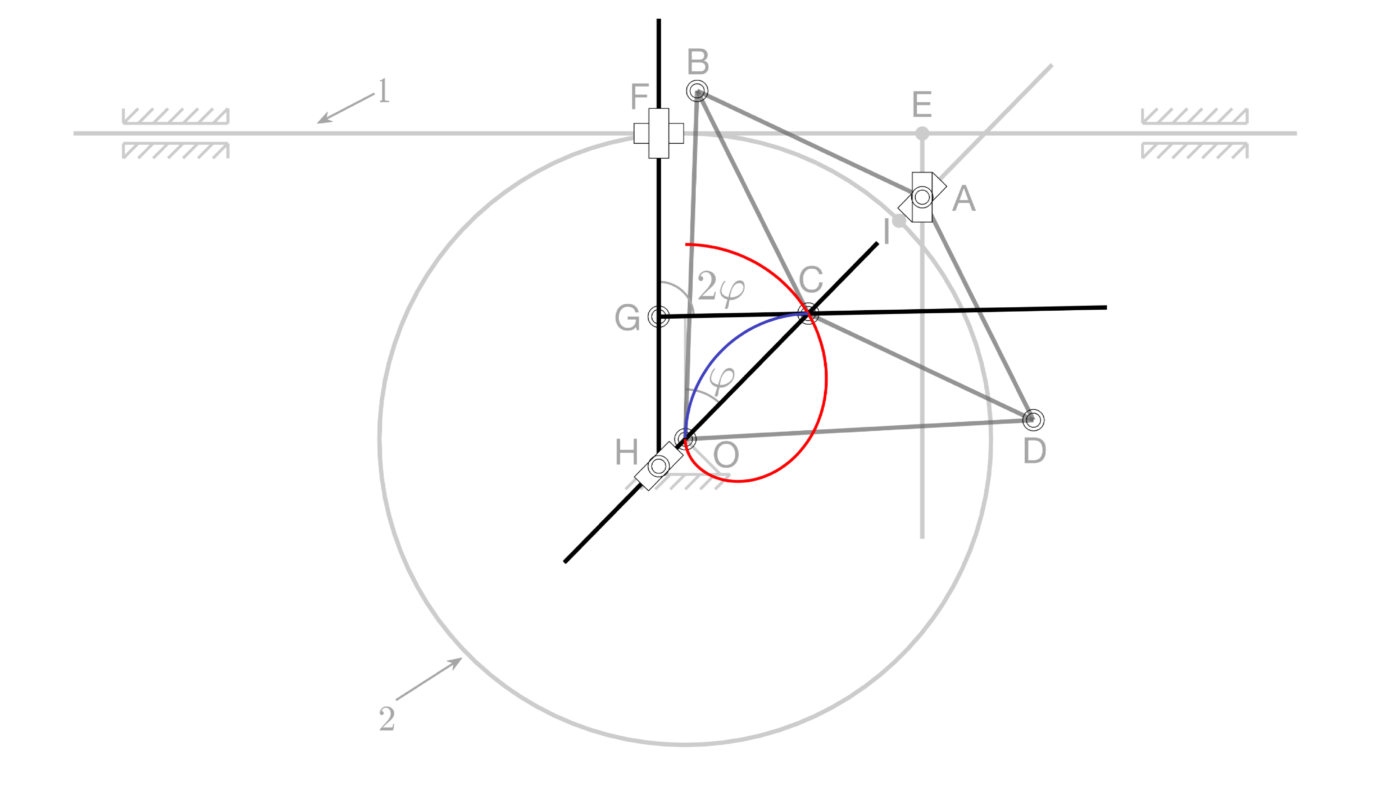
- Exact and approximate mechanisms for pure bending of sheets
Yu, Hanna
[arXiv:1905.07484] | Mechanism and Machine Theory 149 103805 (2020)
Cochleoids and other plane geometry relevant to studies of pleating, folding, creasing, and other forming operations.
- Contrasting bending energies from bulk elastic theories
Wood, Hanna
[arXiv:1808.01310] | Soft Matter 15 2411 (2019) -
Some observations on variational elasticity and its application to plates and membranes
Hanna
[arXiv:1807.06426] | Zeitschrift für angewandte Mathematik und Physik 70 76 (2019)
A long paper resolving and raising some issues that have been bothering me for a while, and a short paper expanding a point from the long paper, in praise of Biot strains for thin structures (please note that the footnote mentioning neo-Hookean energy is half wrong).

- Overcurvature induced multistability of linked conical frusta: How a 'bendy straw' holds its shape
Bende, Yu, Corbin, Dias, Santangelo, Hanna, Hayward
[arXiv:1808.02450] | Soft Matter 14 8636 (2018)
A bendy straw, which can stably adopt just about any one-dimensional shape, only works because it's pre-stressed. Cut one length-wise and watch it relax to a larger radius. An incompatible four-bar linkage works similarly.

- Impact-induced acceleration by obstacles
Corbin, Hanna, Royston, Singh, Warner
[arXiv:1712.05778] | New Journal of Physics 20 053031 (2018) - Chain coiling and impinging
Corbin, Hanna, Royston, Singh, Warner
Gallery of Fluid Motion submission 2017
A chain dropped onto a table falls faster than it would if the table were not there.
- Partial constraint singularities in elastic rods
Hanna, Singh, Virga
[arXiv:1711.00900] | Journal of Elasticity 133 (1) 105-118 (2018)
A classical approach to sleeve constraints, peeling, and similar problems.


- Bifurcations of buckled, clamped anisotropic rods and thin bands under lateral end translations
Yu, Hanna
[arXiv:1708.05968] | Journal of the Mechanics and Physics of Solids 122 657 (2018)
Fun with SO(3). Multi-stability. Snapping movies. Unexpected problems with strip models.
- On the planar elastica, stress, and material stress
Singh, Hanna
[arXiv:1706.03047] | Journal of Elasticity 136 (1) 87 (2019)
An old problem revisited. Classification of elastica by momentum and pseudomomentum.
- Pick-up and impact of flexible bodies
Singh, Hanna
[arXiv:1611.03332] | Journal of the Mechanics and Physics of Solids 106 46-59 (2017) - Chain separation bubbles
Hanna, Royston, Warner
Gallery of Fluid Motion submission 2014
The paper is about contact discontinuities in extended bodies, particularly how the geometry depends on whether you are picking something up or laying it down. Examples include peeling tapes, moored structures, and some musical instruments. The video is an example of two propagating contact discontinuities.
- Catenaries in viscous fluid
Chakrabarti, Hanna
[arXiv:1509.01282] | Journal of Fluids and Structures 66 490-516 (2016)
The classical catenaries are a one-parameter family of curves that don't change shape under translation and axial flow. Stick them in a fluid and they do, picking up four additional shape parameters. These solutions include towing, sedimentation, and other problems as subcases.
- A conserved quantity in thin body dynamics
Hanna, Pendar
[arXiv:1505.05828] | Physics Letters A 380 707-711 (2016)
This note is about a constant arising in dynamical equilibria of thin bodies, which we later learned is related to the Eshelby tensor and to conservation of Kelvin circulation (we now realize that our comparison with the Bernoulli equation is misleading, as it relied on specifics of this problem, and is not generally correct). We also use symmetry arguments to provide the solutions for rotating, flowing strings, such as the steady state of a piece of unspooling yarn.
- Jump conditions for strings and sheets from an action principle
Hanna
[arXiv:1406.5175] | International Journal of Solids and Structures 62 239-247 (2015)
Phenomena such as kinks, shocks, partial contact, cracking, tearing, and cutting of thin structures involve propagating discontinuities in geometry or applied forces. This was my first clumsy attempt to understand these things in terms of action principles for non-material volumes. Some aspects of the approach probably need correction before it can be extended.
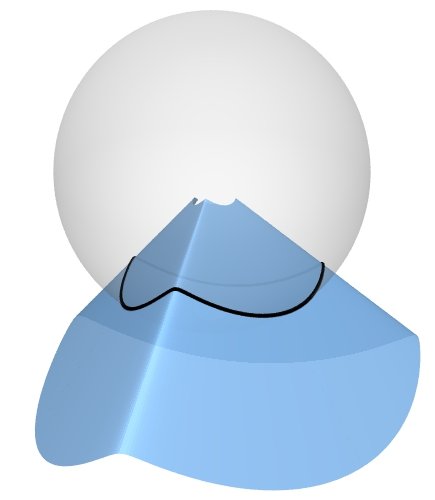
- Whirling skirts and rotating cones
Guven, Hanna, Müller
[arXiv:1306.2619] | Journal of Physics A 46 235201 (2013)
Here we examine the rotation of a flowing, flexible membrane. We find that a minimal model of metrically constrained dynamics generates peaked waves, just like this physical process.

- Dipoles in thin sheets
Guven, Hanna, Kahraman, Müller
[arXiv:1212.3262] | European Physical Journal E 36 106 (2013)
In a sheet of paper, a cone is a monopole: a point singularity in intrinsic curvature. So what's a dipole? There are actually two kinds.
- Unwrapping chains
Cambou, Gamari, Hamm, Hanna, Menon, Santangelo, Walsh
[arXiv:1209.0481] Gallery of Fluid Motion submission 2012
At the free end of a moving string, the stress has to vanish, and a result is that the motion of the end is nontrivial.
- Rotating strings
Hanna
[arXiv:1204.4941] | Journal of Physics A 46 235201 (2013)
These are the three-dimensional "jumpropica" or rigidly rotating strings, generalizing the known planar curves.
- Slack dynamics on an unfurling string
Hanna, Santangelo
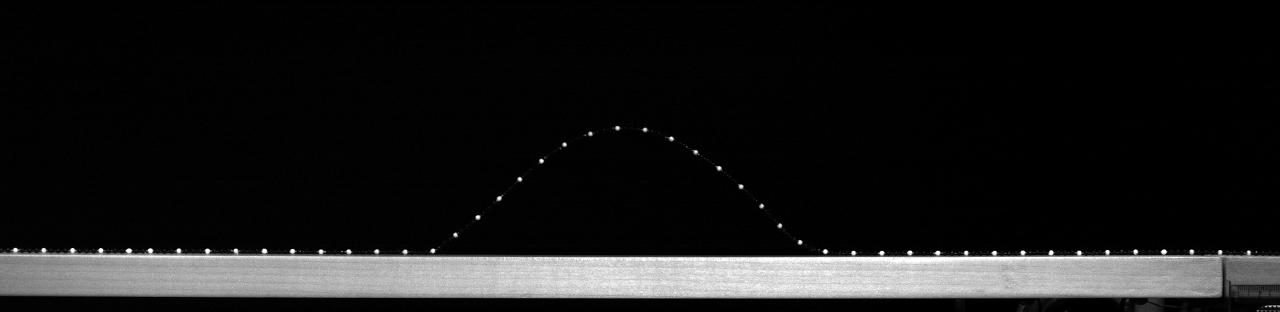
[arXiv:1202.0795] | Physical Review Letters 109 134301 (2012) - An instability in a straightening chain
Hanna, King
[arXiv:1110.2360] Gallery of Fluid Motion submission 2011
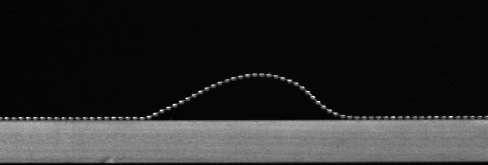
Take a pile of chain or rope sitting on a surface, and pull one end really fast. A strange arch-like feature appears. Our paper talks about several interesting issues that came up while we thought about this problem, though these are not necessarily the most important effects behind the arch and related phenomena such as the "chain fountain", about which open questions remain.

- Designing responsive buckled surfaces by halftone gel lithography
Kim, Hanna, Byun, Santangelo, Hayward
sorry, no arXiv | Science 335 (6073) 1201-1205 (2012)

- Thermally responsive rolling of thin gel strips with discrete variations in swelling
Kim, Hanna, Hayward, Santangelo
sorry, no arXiv | Soft Matter 8 (8) 2375-2381 (2012)
Adventures in making 3D curved surfaces by swelling flat 2D sheets.

- Programmed buckling by controlled lateral swelling in a thin elastic sheet
Dias, Hanna, Santangelo
[arXiv:1105.0704] | Physical Review E 84 036603 (2011)
The original draft had a better title, which you can still see thanks to the arXiv.